Salt Model

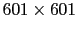Prism wave RTM can be used to delineate the vertical boundaries of a salt flank. In the velocity model shown in Figure (a), an irregular salt body is placed along the left boundary. The model size is $601 \times 601$ points with a 10 m grid interval. The seismic survey contains 301 shots fired at a depth of 10 m with an even $x$ -sampling of 20 m. Every shot is recorded with 601 receivers at a 10 m depth and a 10 m receiver interval along the $x$ -axis. In this case, the velocity gradient is not strong enough to generate diving waves for the short recording aperture of a 6 km long receiver array. 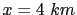Figure  shows a shot gather with the source position at $x=4~km$ , where the prism waves are marked by the yellow arrows.

The 301 shot gathers are migrated with the smooth migration velocity in Figure (b) by a conventional RTM method, and the result is shown in Figure (a). This image clearly illuminates the subhorizontal reflectors, but only a few diffractors are visible along the salt flank. If the subhorizontal reflectors are picked from the RTM image and embedded in the velocity model (Figure (a)), the conventional RTM method can correctly migrate the prism waves to illuminate the steeply dipping salt flank shown in Figure (b). One problem is that the sharp boundaries in the velocity model cause the wavefield to be complex, e.g., internal multiples, and produce artifacts in the RTM image (Figure (b)). Another problem is that the subvertical reflectors are of weaker amplitudes compared to the horizontal ones.

Figure 4.9: (a) A velocity model with a salt body on the left side; (b) the smooth migration velocity model without the salt body.

Figure: A shot gather with the source at 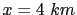$x=4~km$ . The yellow arrows point out the prism waves.

Figure 4.11: (a) The velocity model with subhorizontal reflectors embedded; (b) the RTM image obtained with the velocity model in panel (a). The irregular salt boundary is well imaged.

The prism wave migration method uses the smooth migration velocity (Figure (b)) and the conventional RTM image (Figure (a)) to image the salt flank so that modification of the migration velocity is avoided. Figure (b) shows the prism wave migration image, where the salt flank is clearly imaged with strong amplitudes. However, this image contains some strong artifacts associated with those in Figure (a).

To further improve the image quality, I apply a dip filter to Figure (a) to keep only the subhorizontal reflectors, and the result is shown in Figure (a). Then, the proposed method is applied with the filtered image and the smooth velocity model to migrate the prism waves to produce the image in Figure (b), which contains fewer artifacts compared to Figure (b). Figure (a) shows the image in Figure (b) after dip filtering to keep only the subvertical reflectors. The final image is produced by summation of the migration images in Figures (a) and (a) to give Figure (b), which is the migration image with the best quality.

Figure 4.12: (a) The RTM image obtained with the smooth migration velocity model. Along the salt boundary, only a few diffractors are visible. (b) The RTM image of the prism waves with the same velocity model. The irregular salt boundary is well imaged.

Figure 4.13: (a) The RTM image obtained with the smooth migration velocity model after dip filtering to keep subhorizontal reflectors only; (b) the RTM image of the prism waves.

Figure 4.14: (a) The RTM image of the prism waves after dip filtering for subvertical reflectors only; (b) the sum of two partial images: one from conventional RTM and one from migration of the prism waves.