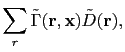


Next: Least-squares Phase-encoded GDM
Up: Theory
Previous: Phase-encoded Reverse-time Migration
Contents
Phase-encoded GDM is equivalent to the phase-encoded RTM but is implemented in a different order; i.e.,
where
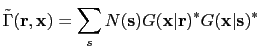 |
(23) |
is the convolution of the receiver-side Green's function and the source-side Green's function followed by
phase-encoding.
It is also known as the multisource migration operator or multisource focusing kernel (Schuster, 2002).
Ge Zhan
2013-07-08
![$\displaystyle \sum_r \sum_s
G({\bf {x}}\vert{\bf {r}})^* \tilde{D}({\bf {r}})
[N({\bf {s}})G({\bf {x}}\vert{\bf {s}})]^* ,$](img190.png)
![$\displaystyle \sum_r \bigg[ \sum_s \overbrace{ N({\bf {s}})G({\bf {x}}\vert{\bf...
...^*G({\bf {x}}\vert{\bf {s}})^*}^{migration~kernel} \bigg] \tilde{D}({\bf {r}}),$](img201.png)