Next: Reflection-Transmission Up: Model Resolution Equation: Previous: Reflection-Migration Contents
More rigorously, Appendix F shows that the model resolution equation 4.6 for diving waves can be transformed into the Fourier integral
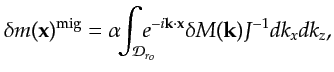 |
(4.10) |
The range of allowable source-geophone
pairs (see Sheng and Schuster (2003)) in
![]() is illustrated in Figure 4.6b,
where only the sources between the blue and red stars
will contribute to the slowness update around the scatterer point at
is illustrated in Figure 4.6b,
where only the sources between the blue and red stars
will contribute to the slowness update around the scatterer point at
![]() .
This differs from the Fourier integral 4.9 for diffraction imaging where
.
This differs from the Fourier integral 4.9 for diffraction imaging where ![]() source-geophone
pairs contribute to the integration domain in
source-geophone
pairs contribute to the integration domain in
![]() for a recorded diffraction. Hence,
the resolution limits for migrating transmission residuals
with the kernel
for a recorded diffraction. Hence,
the resolution limits for migrating transmission residuals
with the kernel
![]() should be worse than migrating diffraction residuals with the same kernel.
should be worse than migrating diffraction residuals with the same kernel.
![\includegraphics[width=4.5in]{figs/wavepath1/Rabbit2}](img431.png) |
The precise connection between intersecting wavepaths in Figure 4.2b, the range of available wavenumbers,
and resolution limits in equation 4.2
can be made by assuming a homogeneous medium.
In this case,
Figure 4.7 shows that the
half-width ![]() of the first Fresnel zone at the point midway
between the source and geophone is equal to
of the first Fresnel zone at the point midway
between the source and geophone is equal to
For a single source-geophone pair, the
best direction of transmission spatial resolution
for a slowness anomaly midway between the
source and geophone is perpendicular to the central ray.
This means that a slowness anomaly moved perpendicular to the
ray from
the central ray will lead to the most noticeable change in the transmission arrival. The worst direction of spatial resolution
is along the ray itself because the slowness anomaly can be slid
along it without changing the traveltime; moreover, the model
wavenumber
![]() is zero all along the transmission central ray.
is zero all along the transmission central ray.
Yunsong Huang 2013-09-22